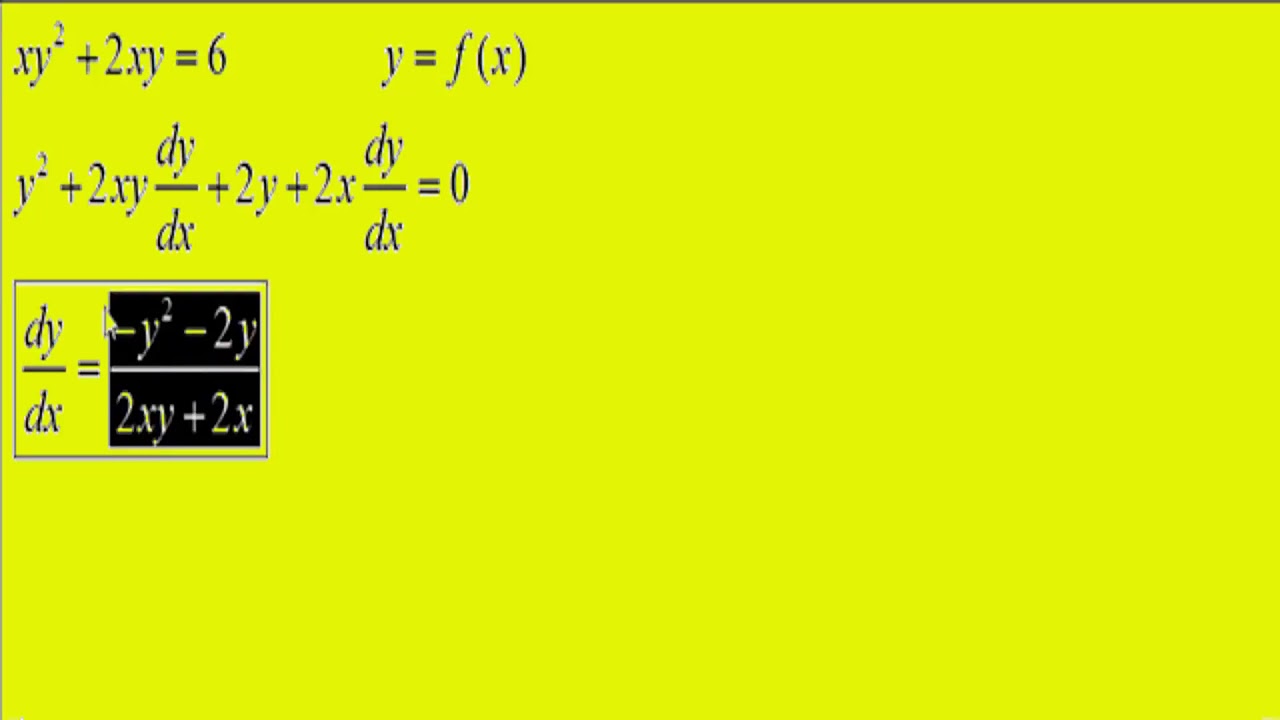Mastering Implicit Differentiation: The Ultimate Implicit Differentiation Calculator

Implicit differentiation can often appear daunting, especially for students and professionals trying to navigate the complexities of calculus. However, the advent of an implicit differentiation calculator has immensely simplified the process, allowing users to tackle intricate equations with ease. By understanding the principles behind implicit differentiation, learners can gain a deeper appreciation for the beauty of calculus and its applications in various fields. The ability to differentiate equations that are not explicitly solved for one variable in terms of another opens up a world of possibilities. This is particularly useful in fields such as physics, engineering, and economics, where relationships between variables are often intertwined.

With an implicit differentiation calculator at your fingertips, you can avoid tedious manual calculations while ensuring accuracy in your results. This tool not only saves time but also reduces the likelihood of errors that can occur during the differentiation process. As you delve deeper into the world of calculus, the importance of mastering these concepts becomes increasingly clear, empowering you to tackle complex problems with confidence.

Whether you're a student preparing for exams or a professional looking to refine your mathematical skills, understanding how to use an implicit differentiation calculator can significantly enhance your problem-solving toolkit. In this article, we will explore the ins and outs of implicit differentiation, how to effectively use a calculator for this purpose, and answer some common questions associated with the topic. Get ready to unlock the secrets of implicit differentiation!

What is Implicit Differentiation?

Implicit differentiation is a technique used in calculus to differentiate equations that define a relationship between two or more variables without explicitly solving for one variable in terms of another. Unlike explicit differentiation, where a function is clearly defined (e.g., y = f(x)), implicit differentiation is applicable to equations that may involve variables intertwined in a more complex way (e.g., F(x, y) = 0).

Why Use Implicit Differentiation?

Implicit differentiation is particularly useful in situations where it's difficult, if not impossible, to isolate one variable. Here are a few reasons to employ this method:

• Handles complex relationships between variables
• Avoids cumbersome algebraic manipulation
• Facilitates the derivation of slopes for curves defined by implicit functions

How Does an Implicit Differentiation Calculator Work?

An implicit differentiation calculator automates the process of differentiating equations by applying the necessary rules of calculus. Users simply input the equation they wish to differentiate, and the calculator performs the steps to find the derivative with respect to the desired variable. This tool simplifies the process and provides immediate results, making it an invaluable resource for students and professionals alike.

What Steps Are Involved in Using an Implicit Differentiation Calculator?

Using an implicit differentiation calculator is straightforward. Here are the general steps:

1. Enter the equation in the provided input field.
2. Select the variable to differentiate with respect to (e.g., x or y).
3. Click on the calculate button to obtain the derivative.

What Are the Common Challenges in Implicit Differentiation?

Despite the utility of implicit differentiation, students often encounter challenges such as:

• Confusion regarding the application of the chain rule
• Difficulty in managing multiple variables
• Errors in algebraic manipulation

How Can I Overcome These Challenges?

Overcoming challenges in implicit differentiation involves practice and familiarity with calculus principles. Here are some tips:

• Practice solving various implicit equations
• Review the chain rule and its applications
• Utilize an implicit differentiation calculator to verify manual calculations

Can Implicit Differentiation Be Applied in Real-World Scenarios?

Implicit differentiation is not just theoretical; it has practical applications across various fields. Some examples include:

• Physics: Analyzing trajectories of moving objects
• Engineering: Designing curves and surfaces
• Economics: Modeling relationships between different economic variables

What Are Some Examples of Implicit Differentiation?

To further understand implicit differentiation, let’s look at a couple of examples:

• Example 1: For the equation x^2 + y^2 = 1 (the equation of a circle), the derivative dy/dx can be found through implicit differentiation.
• Example 2: For the equation xy + y^2 = 3, differentiating both sides leads to an expression for dy/dx that reflects the relationship between x and y.

Conclusion: Why You Should Embrace the Implicit Differentiation Calculator

Understanding and applying implicit differentiation is a vital skill in calculus. The implicit differentiation calculator serves as an excellent tool to simplify this process, allowing users to focus on understanding concepts rather than getting bogged down by calculations. By embracing this calculator, you can enhance your mathematical prowess and tackle complex problems with greater ease. So, why wait? Start exploring the world of implicit differentiation with the help of this powerful tool today!